Skewed Curves
Skewed curves are asymmetrical curves; their skewness is caused by “outliers.” (An outlier is a number that’s much smaller or much larger than all other numbers in a data set.) One or just a few outliers, in a data set, can cause these curves have a “tail.” Data is not normally distributed in skewed curves.
USMLE high yield concepts include knowing, for example, if the mean is less than or more than the mode when a curve is skewed positively. Or, what happens to the mean, median and mode if the largest number in a data set is removed (i.e., if an outlier is removed)? If you can count, “1, 2, 3,” then the USMLEbiostatistics workbook offers the easiest 3 Steps imaginable so you will NEVER miss a question about skewed curves.
Core concepts of skewed curves
Skewed curves are asymmetrical curves: they “skew” negatively (the tail points left) or positively (the tail points right). Skewed curves NEVER have the mean, median & mode in the same location. This is distinctly different than the bell curve, which is symmetrical.
Also, a negatively skewed curves can be of entirely positive numbers and, positively skewed curves can be of entirely negative numbers. “Positive” and “negative” provides you the direction of the curve’s tail and, the direction that numbers are moving on the x-axis.
Negative skew: points in negative direction
numbers on the x-axis, under the tail, are less than the numbers under the hump; negatively skewed curves do NOT necessarily have negative numbers (as in example below)
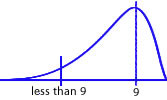
Positive skew: points in positive direction
numbers on the x-axis, under the tail, are less than the numbers under the hump; negatively skewed curves do NOT necessarily have negative numbers (as in example below)

Answering high yield skewed curve concept questions
I don’t see an answer that would require a calculation; instead, it’s entirely conceptual. Again, if you can count to 3 then you will NEVER miss a skewed curve question — it’s that easy! No matter which way they try to fool you, 1 < 2 < 3.
But another tricky question: what happens to the mode, median & mean, of a negatively skewed curve, if smallest number is removed. How would the mode, median & mean be affected?
Would the
mean increase, decrease or not change?
median increase, decrease or not change? and,
mode increase, decrease or not change?
The USMLEbiostatistics workbook will provide the no-brainer 3 Step process, provide additional details, offer a wide variety of word-problems with thorough explanations, and much more. Buy now!